.
MONEY
WHAT IT IS
HOW IT WORKS
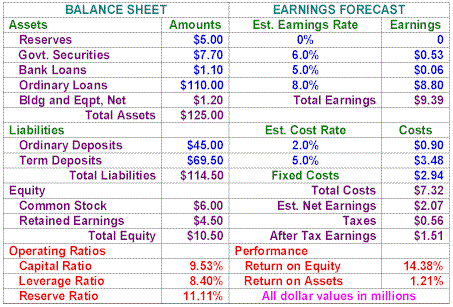
A good way to understand how banks work is to imagine starting your own bank. The first thing you need to do is put up some of your own money. You won’t receive a banking license unless you have your own capital at risk. Getting Started Let’s assume you raise $6 million in cash with help from other investors. That will be the bank’s initial equity, the owner’s stake. Next you obtain a charter, rent a building, furnish it with all the necessary equipment, hire and train a staff, and open your doors for business. You’ll need to deposit some of your initial stake at the Fed. Those funds will be used to clear checks written by your own depositors. You’ll also need to keep enough cash in the vault to meet the demand for withdrawals by your depositors. Let's assume initial expenses of $1.2 million. That leaves $4.8 million, of which you allocate $2 million to vault cash and $2.8 million to your Fed account. Managing Loans and Other Investments As your business develops, some customers will deposit their own money to open checking accounts. Others will invest in your savings accounts and certificates of deposit (term loans) which must pay a competitive interest rate. Still others will seek loans from the bank. It is up to you to determine whether prospective borrowers are good credit risks, and will be able to pay the interest charges and return the principal on the specified date. Accounting Needs In managing your bank, you will need an accounting system to determine how your decisions are likely to affect the bank’s profitability. The most important account is the balance sheet. This shows at any given moment, the bank’s assets (what it owns), its liabilities (what it owes to others), and its net worth (what belongs to the owners). Net worth, or equity, is equal to assets minus liabilities. Your equity should remain positive and preferably growing. If it ever gets too low relative to total assets, your regulator may close the bank. Balance Sheet and Earnings Forecasts If your bank does well, the balance sheet will expand with new assets and liabilities. The equity should also increase, assuming you retain some of the profits in the bank rather than pay them all out as dividends to the owners. You started with initial equity of $6 million. Let’s take a look at the balance sheet after you have been in business for some time. It is shown together with an earnings forecast for the coming year.
The earnings forecast is based on expected earning rates of the bank’s assets and the cost of borrowed funds. Also shown is the expected cost of operations or fixed costs, covering rent, insurance, utilities, salaries, etc. The entries in blue are items that you might try to modify to see how they would affect the key performance measure, the return on equity. Of course, you must maintain the required minimum ratios set by the regulators. Growth Management Note that your equity has grown from $6.0 million to $10.5 million due to retained earnings. You have acquired a substantial amount in deposits, some of which are ordinary checking accounts that pay no interest. Others were borrowed at market rates. All deposits whether or not they bear interest have associated costs. With the additional funds available from deposits, you have redistributed your assets to what you hope will enhance future earnings: $5.0 million in reserves, $7.7 million in T-bills, $1.1 million in loans to other banks, and $110 million in ordinary loans. You project net earnings for the coming year after taxes of $1.51 million. That would be a return on equity of 14.38% and a return on assets of 1.21%, which is quite reasonable performance. Required Operating Ratios In the lower left corner of the table are the three ratios that must be kept above minimum values established by bank regulators. The capital ratio is the ratio of a bank’s equity to a risk-weighted sum of the bank's assets. The weightings are 0 for reserves, 0 for government securities, 0.2 for loans to banks, and 1.0 for ordinary loans. A minimum capital ratio of 8% is required. The leverage ratio is the ratio of a bank's equity to the unweighted sum of its total assets. The required minimum is 3%. The reserve ratio is the ratio of a bank's reserves (deposits at the Fed plus vault cash) to its demand deposits, i.e. checking deposits. The required minimum is 10% for large banks, but only 3% on the first $45.4 million of demand deposits, which is the case for your small bank. How Transactions Affect Operating Ratios When a bank issues an ordinary loan, its assets (A) and liabilities (L) increase equally, while its reserves (R) remain unchanged. The reserve ratio (R/L) decreases due to the increase in L. Capital (C = A - L) remains unchanged, and the capital ratio (C/A) decreases due to the increase in A. When computing the capital ratio, we use the risk-weighted value of A in the denominator. In this case, the increase in A is all due to an increase in ordinary loans which have a risk weighting of 1.0. When the borrower spends the funds, if they are deposited in another bank, R and L decrease by the same amount. Since R is typically a small fraction of L, R/L decreases. Risk-weighted assets remain unchanged because the only component of A that changes is R which has a risk-weighting of 0. Therefore C/A remains unchanged. If the spent funds are deposited in the bank making the loan, R, L, and A remain unchanged. so there is no change in R/L and C/A. When the borrower pays interest on the loan out of a deposit within the bank, L decreases while A and R remain unchanged. This results in an increase in R/L due to the decrease in L, and an increase in C/A due to the increase in C. If the borrower pays interest from an outside source, A and R increase while L remains unchanged. This results in an increase in R/L due to the increase in R, and an increase in C/A due to the increase in C since risk-weighted assets remain unchanged. When the borrower repays the loan from a deposit within
the bank, R
remains unchanged while A and L
decrease equally. R/L increases due to the decrease in L, and C/A remains unchanged. If the borrower repays the loan from an external source, R increases and L remains unchanged causing an increase in R/L. Total assets remain unchanged because the decrease in loan assets is offset by the increase in R. However the decrease in loan assets
decreases risk-weighted assets and therefore increases the C/A. When a bank pays for its operating expenses, it may issue a bank draft. If the recipient deposits the draft in the same bank, he receives a deposit which increases L, while A and R remain unchanged. If he deposits it in another bank, A and R decrease while L remains unchanged. In both cases, the capital ratio and reserve ratio of the issuing bank decrease. While there is much more to learn about banks, this
simplified model
outlines the essential details for small banks. However large
banks
are far more complex institutions. For some of them,
lending
is a minor part of the business. The next article surveys the
main
activities of large banks. |